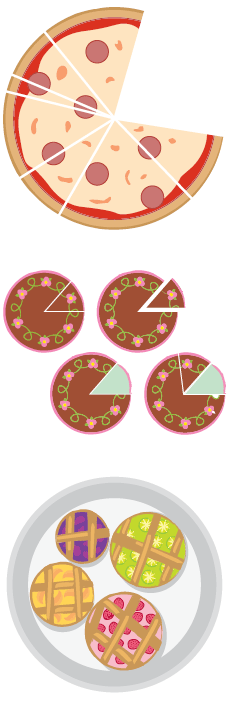
- Two hungry Icelanders, Alta and Baldur, are sharing a pizza that is radially sliced into pieces of various sizes. To start, Alta chooses any slice to eat; thereafter, she and Baldur alternate taking slices (Baldur first) but must always take a slice that is adjacent to some previously taken slice. I call this the "polite pizza protocol"; the uneaten portion is always connected, thus, in theory, staying hot longer. Is it possible for the pizza to be cut in such a way that, no matter what Alta does, Baldur can get more than half the pie?
- A cylindrical ice-cream cake with the most scrumptious chocolate frosting on top is sitting on a table. As an expert cake cutter, you choose an arbitrary angle x and proceed to cut one wedge after another, counterclockwise, around the cake, each of angle exactly x. However, each time you cut a wedge, you turn that piece upside-down and slide it back into the cake. This puts the frosting on the bottom at first, but as you work your way around and around the cake, the frosting comes back up to the top, then returns to the bottom, and so forth. Your mission is to prove that after some finite number of slices, all the frosting will be back on top of the cake.
- You need to bake circular tarts of various sizes and total area 1. Can you always fit them into a circular pie pan of area 2? Amazingly, no one knows.
(This puzzle is from Alexander Soifer of the University of Colorado, Colorado Springs; related work, including a proof for square tarts in a square pan, can be found in the journal Geombinatorics, www.uccs.edu/~geombina/.)
Last byte
Puzzled: Circular Food
Welcome to three new challenging mathematical puzzles. Solutions to the first two will be published next month; the third is as yet unsolved, so you may need extra luck with that one. Here, I concentrate on circular food, so you might want to eat something before jumping in.
The Latest from CACM
Shape the Future of Computing
ACM encourages its members to take a direct hand in shaping the future of the association. There are more ways than ever to get involved.
Get InvolvedCommunications of the ACM (CACM) is now a fully Open Access publication.
By opening CACM to the world, we hope to increase engagement among the broader computer science community and encourage non-members to discover the rich resources ACM has to offer.
Learn More

Join the Discussion (0)
Become a Member or Sign In to Post a Comment